Frequency of electromagnetic oscillations formula. Electromagnetic vibrations. Harmonic law of oscillations in a circuit
- Electromagnetic vibrations– these are periodic changes over time in electrical and magnetic quantities in an electrical circuit.
- Free these are called fluctuations, which arise in a closed system as a result of deviation of this system from a state of stable equilibrium.
During oscillations, a continuous process of converting the energy of the system from one form to another occurs. In the case of oscillations of the electromagnetic field, exchange can only take place between the electric and magnetic components of this field. The simplest system where this process can occur is oscillatory circuit.
- Ideal oscillatory circuit (LC circuit) - an electrical circuit consisting of an inductive coil L and a capacitor with a capacity C.
Unlike a real oscillatory circuit, which has electrical resistance R, the electrical resistance of an ideal circuit is always zero. Therefore, an ideal oscillatory circuit is a simplified model of a real circuit.
Figure 1 shows a diagram of an ideal oscillatory circuit.
Circuit energies
Total energy of the oscillatory circuit
\(W=W_(e) + W_(m), \; \; \; W_(e) =\dfrac(C\cdot u^(2) )(2) = \dfrac(q^(2) ) (2C), \; \; W_(m) =\dfrac(L\cdot i^(2))(2),\)
Where W e- energy of the electric field of the oscillatory circuit at a given time, WITH- electrical capacity of the capacitor, u- the voltage value on the capacitor at a given time, q- value of the capacitor charge at a given time, Wm- energy of the magnetic field of the oscillatory circuit at a given time, L- coil inductance, i- current value in the coil at a given time.
Processes in an oscillatory circuit
Let us consider the processes that occur in an oscillatory circuit.
To remove the circuit from the equilibrium position, we charge the capacitor so that there is a charge on its plates Qm(Fig. 2, position 1 ). Taking into account the equation \(U_(m)=\dfrac(Q_(m))(C)\) we find the voltage value on the capacitor. There is no current in the circuit at this moment in time, i.e. i = 0.
After closing the key under the influence of the electric field of the capacitor, an electric current will appear in the circuit, the current strength i which will increase over time. The capacitor will begin to discharge at this time, because electrons creating a current (I remind you that the direction of current is taken to be the direction of movement of positive charges) leave the negative plate of the capacitor and come to the positive one (see Fig. 2, position 2 ). Along with charge q tension will also decrease u\(\left(u = \dfrac(q)(C) \right).\) When the current strength increases through the coil, a self-induction emf will arise, which prevents the current from changing. As a result, the current strength in the oscillating circuit will increase from zero to a certain maximum value not instantly, but over a certain period of time determined by the inductance of the coil.
Capacitor charge q decreases and at some point in time becomes equal to zero ( q = 0, u= 0), the current in the coil will reach a certain value I m(see Fig. 2, position 3 ).
Without the electric field of the capacitor (and resistance), the electrons creating the current continue to move by inertia. In this case, electrons arriving at the neutral plate of the capacitor impart a negative charge to it, and electrons leaving the neutral plate impart a positive charge to it. A charge begins to appear on the capacitor q(and voltage u), but of the opposite sign, i.e. the capacitor is recharged. Now the new electric field of the capacitor prevents the electrons from moving, so the current i begins to decrease (see Fig. 2, position 4 ). Again, this does not happen instantly, since now the self-induction EMF tends to compensate for the decrease in current and “supports” it. And the current value I m(pregnant 3 ) turns out maximum current value in the circuit.
And again, under the influence of the electric field of the capacitor, an electric current will appear in the circuit, but directed in the opposite direction, the current strength i which will increase over time. And the capacitor will be discharged at this time (see Fig. 2, position 6 )to zero (see Fig. 2, position 7 ). And so on.
Since the charge on the capacitor q(and voltage u) determines its electric field energy W e\(\left(W_(e)=\dfrac(q^(2))(2C)=\dfrac(C \cdot u^(2))(2) \right),\) and the current strength in the coil i- magnetic field energy Wm\(\left(W_(m)=\dfrac(L \cdot i^(2))(2) \right),\) then along with changes in charge, voltage and current, energy will also change.

Designations in the table:
\(W_(e\, \max ) =\dfrac(Q_(m)^(2) )(2C) =\dfrac(C\cdot U_(m)^(2) )(2), \; \; \; W_(e\, 2) =\dfrac(q_(2)^(2) )(2C) =\dfrac(C\cdot u_(2)^(2) )(2), \; ; W_(e\, 4) =\dfrac(q_(4)^(2) )(2C) =\dfrac(C\cdot u_(4)^(2) )(2), \; W_(e\, 6) =\dfrac(q_(6)^(2) )(2C) =\dfrac(C\cdot u_(6)^(2) )(2),\)
\(W_(m\; \max ) =\dfrac(L\cdot I_(m)^(2) )(2), \; \; \; W_(m2) =\dfrac(L\cdot i_(2 )^(2) )(2), \; W_(m4) =\dfrac(L\cdot i_(4)^(2), \; =\dfrac(L\cdot i_(6)^(2) )(2).\)
The total energy of an ideal oscillating circuit is conserved over time because there is no energy loss (no resistance). Then
\(W=W_(e\, \max ) = W_(m\, \max ) = W_(e2) + W_(m2) = W_(e4) +W_(m4) = ...\)
Thus, in an ideal L.C.- the circuit will undergo periodic changes in current values i, charge q and voltage u, and the total energy of the circuit will remain constant. In this case, they say that there are problems in the circuit free electromagnetic oscillations.
- Free electromagnetic oscillations in the circuit - these are periodic changes in the charge on the capacitor plates, current and voltage in the circuit, occurring without consuming energy from external sources.
Thus, the occurrence of free electromagnetic oscillations in the circuit is due to the recharging of the capacitor and the occurrence of a self-inductive emf in the coil, which “provides” this recharging. Note that the capacitor charge q and the current in the coil i reach their maximum values Qm And I m at various points in time.
Free electromagnetic oscillations in the circuit occur according to the harmonic law:
\(q=Q_(m) \cdot \cos \left(\omega \cdot t+\varphi _(1) \right), \; \; \; u=U_(m) \cdot \cos \left(\ omega \cdot t+\varphi _(1) \right), \; \; i=I_(m) \cdot \cos \left(\omega \cdot t+\varphi _(2) \right).\)
The shortest period of time during which L.C.- the circuit returns to its original state (to the initial value of the charge of a given plate), called the period of free (natural) electromagnetic oscillations in the circuit.
The period of free electromagnetic oscillations in L.C.-contour is determined by Thomson’s formula:
\(T=2\pi \cdot \sqrt(L\cdot C), \;\;\; \omega =\dfrac(1)(\sqrt(L\cdot C)).\)
From the point of view of mechanical analogy, a spring pendulum without friction corresponds to an ideal oscillatory circuit, and a real one - with friction. Due to the action of friction forces, the oscillations of a spring pendulum fade over time.
*Derivation of Thomson's formula
Since the total energy of the ideal L.C.-circuit equal to the sum of the energies of the electrostatic field of the capacitor and the magnetic field of the coil is conserved, then at any time the equality is valid
\(W=\dfrac(Q_(m)^(2) )(2C) =\dfrac(L\cdot I_(m)^(2) )(2) =\dfrac(q^(2) )(2C ) +\dfrac(L\cdot i^(2) )(2) =(\rm const).\)
We obtain the equation of oscillations in L.C.-circuit using the law of conservation of energy. Differentiating the expression for its total energy with respect to time, taking into account the fact that
\(W"=0, \;\;\; q"=i, \;\;\; i"=q"",\)
we obtain an equation describing free oscillations in an ideal circuit:
\(\left(\dfrac(q^(2) )(2C) +\dfrac(L\cdot i^(2) )(2) \right)^((") ) =\dfrac(q)(C ) \cdot q"+L\cdot i\cdot i" = \dfrac(q)(C) \cdot q"+L\cdot q"\cdot q""=0,\)
\(\dfrac(q)(C) +L\cdot q""=0,\; \; \; \; q""+\dfrac(1)(L\cdot C) \cdot q=0.\ )
Rewriting it as:
\(q""+\omega ^(2) \cdot q=0,\)
we note that this is the equation of harmonic oscillations with a cyclic frequency
\(\omega =\dfrac(1)(\sqrt(L\cdot C) ).\)
Accordingly, the period of the considered oscillations
\(T=\dfrac(2\pi )(\omega ) =2\pi \cdot \sqrt(L\cdot C).\)
Literature
- Zhilko, V.V. Physics: textbook. manual for 11th grade general education. school from Russian language training / V.V. Zhilko, L.G. Markovich. - Minsk: Nar. Asveta, 2009. - pp. 39-43.
Lesson No. 48-169 Oscillatory circuit. Free electromagnetic oscillations. Conversion of energy in an oscillatory circuit. Thompson's formula.Oscillations- movements or states that repeat over time.Electromagnetic vibrations -these are electrical vibrations andmagnetic fields that resistdriven by periodic infidelitycharge, current and voltage. An oscillatory circuit is a system consisting of an inductor and a capacitor(Fig. a). If the capacitor is charged and shorted to the coil, then current will flow through the coil (Fig. b). When the capacitor is discharged, the current in the circuit will not stop due to self-induction in the coil. The induction current, in accordance with Lenz's rule, will flow in the same direction and recharge the capacitor (Fig. c). The current in this direction will stop, and the process will repeat in the opposite direction (Fig. G).
Thus, in fluctuationstelny contour of the originelectromagnetic oscillationsnia due to energy conversionelectric field condensationra( W E = 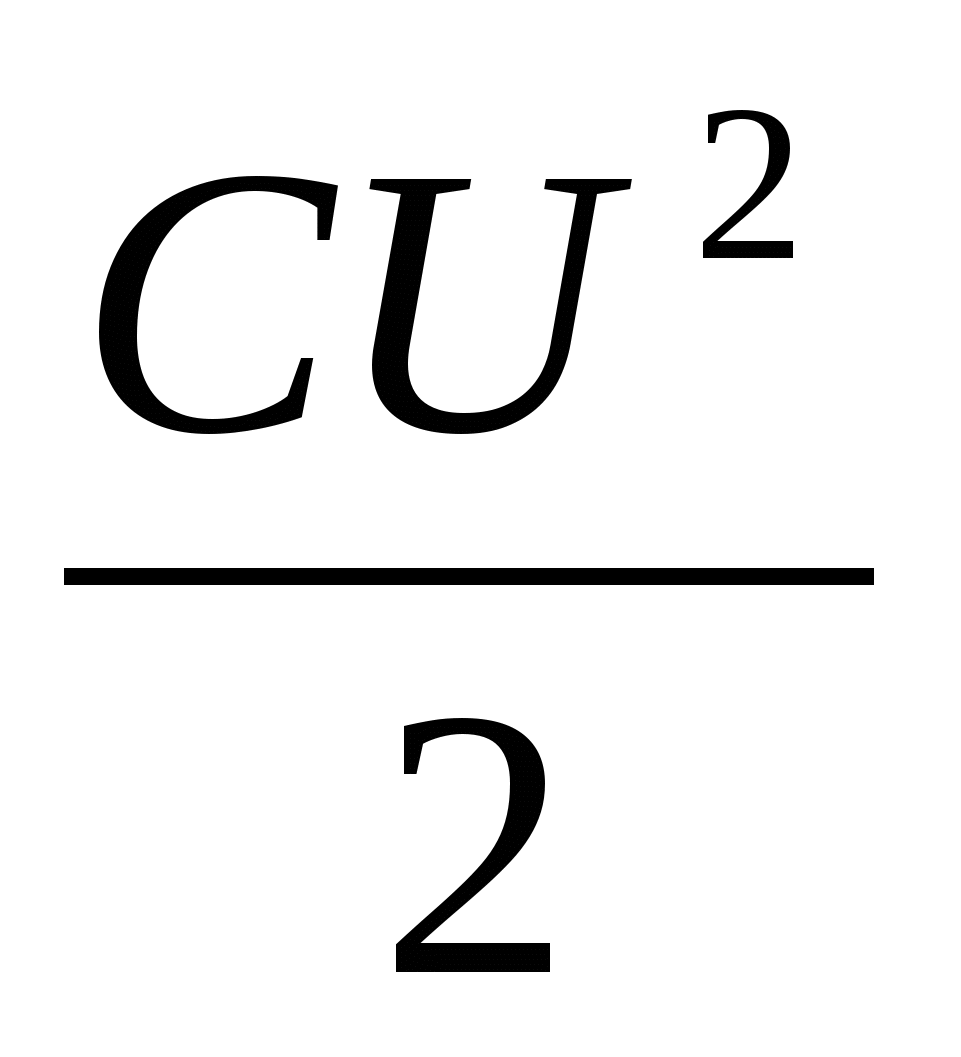 )
into the energy of the magnetic field of a coil with current(W M =
)
into the energy of the magnetic field of a coil with current(W M =  ),
and vice versa.
),
and vice versa.
Harmonic oscillations are periodic changes in a physical quantity depending on time, occurring according to the law of sine or cosine.
 The equation describing free electromagnetic oscillations takes the form
The equation describing free electromagnetic oscillations takes the form
q"= - ω 0 2 q (q" is the second derivative.
Main characteristics of oscillatory motion:
The period of oscillation is the minimum period of time T after which the process is completely repeated.
The amplitude of harmonic oscillations is the modulus of the largest value of the oscillating quantity.
Knowing the period, you can determine the frequency of oscillations, i.e. the number of oscillations per unit of time, for example per second. If one oscillation occurs in time T, then the number of oscillations in 1 s ν is determined as follows: ν = 1/T.
Recall that in the International System of Units (SI), the frequency of oscillations is equal to one if one oscillation occurs in 1 s. The unit of frequency is called the hertz (abbreviated: Hz) after the German physicist Heinrich Hertz.
After a period of time equal to the period T, i.e., when the cosine argument increases by ω 0
T, the charge value is repeated and the cosine takes on its previous value. From the mathematics course we know that the smallest period of the cosine is 2n. Therefore, ω 0
T=2π, whence ω 0
= 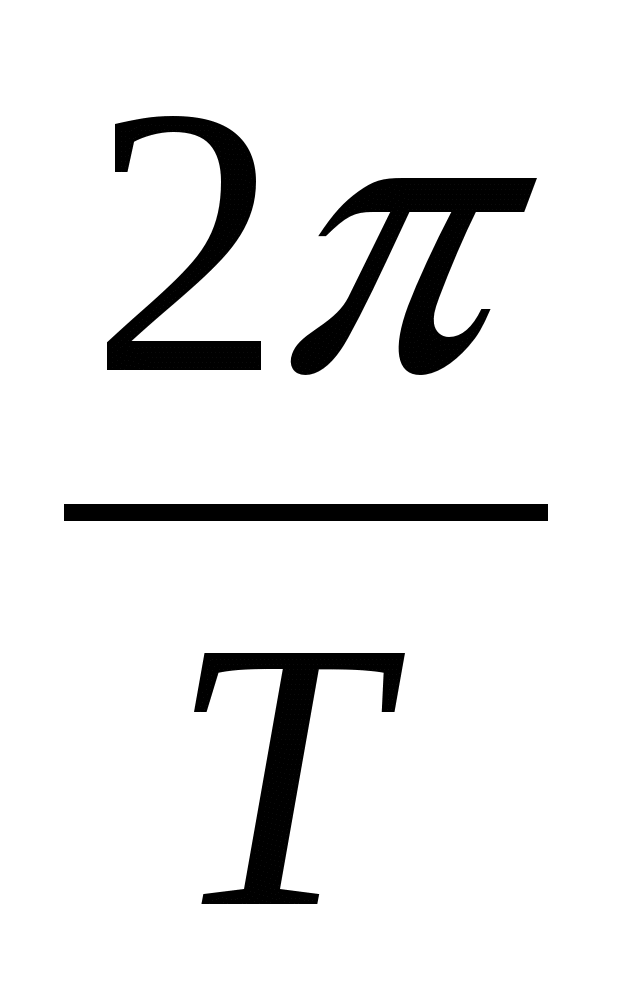 =2πν Thus, the value ω 0
- this is the number of oscillations, but not in 1 s, but in 2 s. It is called cyclical or circular frequency.
=2πν Thus, the value ω 0
- this is the number of oscillations, but not in 1 s, but in 2 s. It is called cyclical or circular frequency.
The frequency of free oscillations is called natural vibrational frequencysystems. Often in the following, for brevity, we will simply refer to the cyclic frequency as frequency. Distinguish cyclic frequency ω 0 from frequency ν can be used according to the notation.
By analogy with the solution of the differential equation for a mechanical oscillatory system cyclic frequency of free electricalsky fluctuations is equal to:ω 0 = 
The period of free oscillations in the circuit is equal to: T=  =2π
=2π 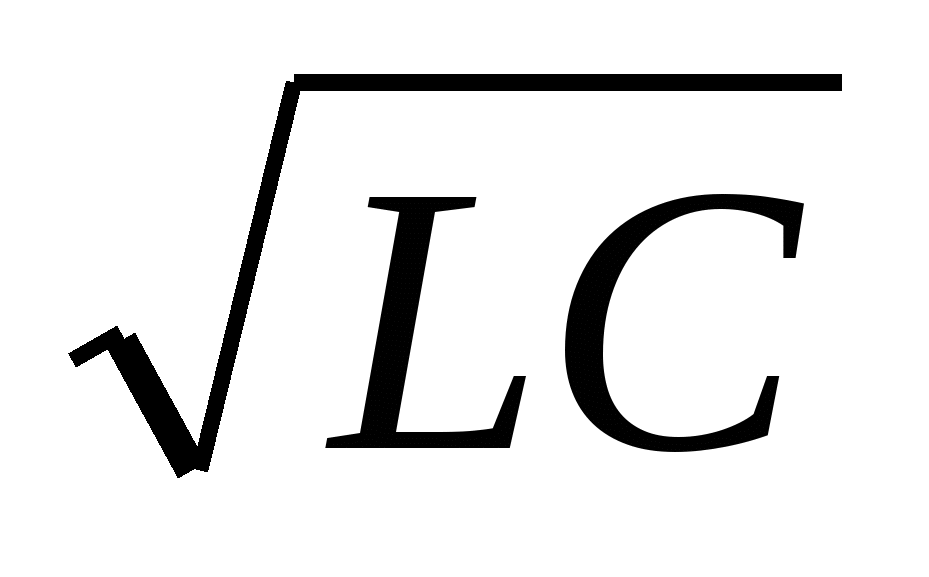 - Thomson's formula.
- Thomson's formula.
The phase of oscillations (from the Greek word phasis - appearance, stage of development of a phenomenon) is the value φ, standing under the sign of cosine or sine. The phase is expressed in angular units - radians. The phase determines, for a given amplitude, the state of the oscillatory system at any time.
Oscillations with the same amplitudes and frequencies may differ from each other in phases.
Since ω 0
= , then φ= ω 0
Т=2π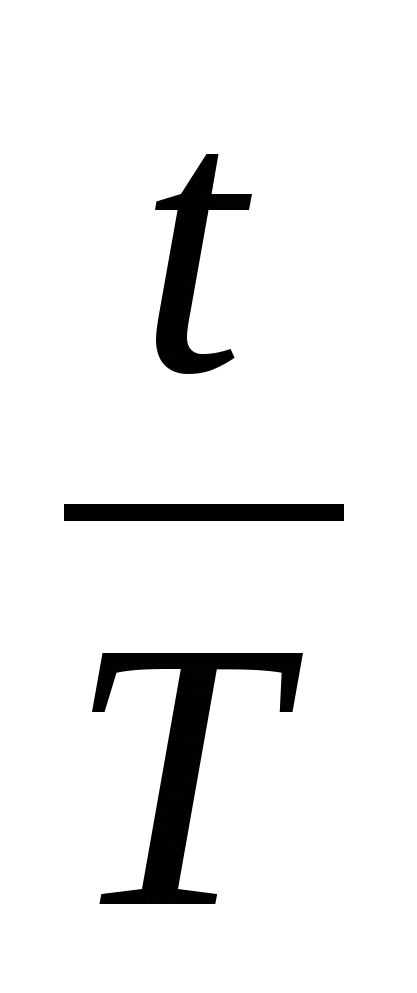 . The ratio shows how much of the period has passed since the start of the oscillation. Any time value expressed in fractions of a period corresponds to a phase value expressed in radians. So, after time t=
. The ratio shows how much of the period has passed since the start of the oscillation. Any time value expressed in fractions of a period corresponds to a phase value expressed in radians. So, after time t= 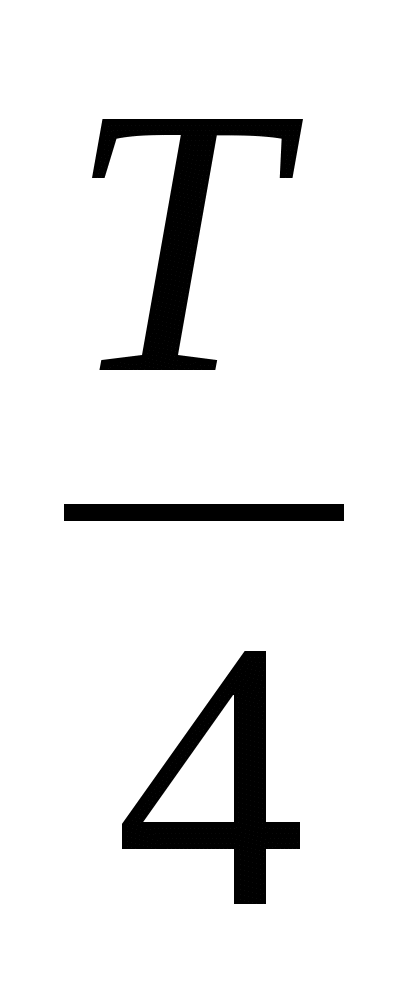 (quarter period) φ=
(quarter period) φ= 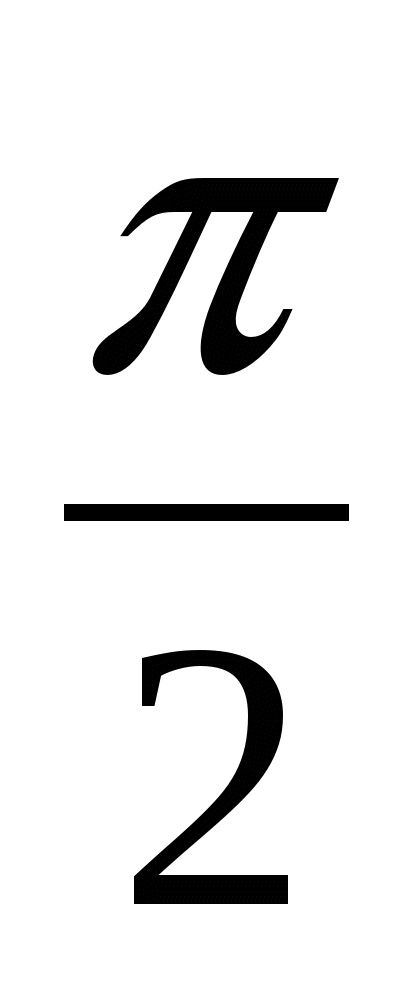 , after half the period φ = π, after the whole period φ = 2π, etc. You can plot the dependence
, after half the period φ = π, after the whole period φ = 2π, etc. You can plot the dependence
 charge does not depend on time, but on phase. The figure shows the same cosine wave as the previous one, but on the horizontal axis it is plotted instead of time
charge does not depend on time, but on phase. The figure shows the same cosine wave as the previous one, but on the horizontal axis it is plotted instead of time
different phase values φ.
Correspondence between mechanical and electrical quantities in oscillatory processes
Mechanical quantities
Tasks.942(932). The initial charge imparted to the capacitor of the oscillatory circuit was reduced by 2 times. How many times did: a) voltage amplitude change; b) current amplitude;
c) the total energy of the electric field of the capacitor and the magnetic field of the coil?
943(933). With an increase in the voltage on the capacitor of the oscillatory circuit by 20 V, the amplitude of the current increased by 2 times. Find the initial voltage.
945(935). The oscillatory circuit consists of a capacitor with a capacity C = 400 pF and an inductance coil L = 10 mH. Find the amplitude of current oscillations I T , if the amplitude of voltage fluctuations U T = 500 V.
952(942). After what time (in fractions of the period t/T) for the first time there will be a charge on the capacitor of the oscillating circuit equal to half the amplitude value?
957(947). What inductance coil should be included in the oscillatory circuit in order to obtain a free oscillation frequency of 10 MHz with a capacitor capacitance of 50 pF?
Oscillatory circuit. Period of free oscillations.
1. After the capacitor of the oscillating circuit has been given a charge q = 10 -5 C, damped oscillations arose in the circuit. How much heat will be released in the circuit by the time the oscillations in it completely die out? Capacitance of the capacitor C = 0.01 μF.
2. The oscillating circuit consists of a capacitor with a capacity of 400 nF and a coil with an inductance of 9 μH. What is the period of natural oscillation of the circuit?
3. What inductance must be included in the oscillatory circuit in order to obtain a natural oscillation period of 2∙ 10 -6 s with a capacitance of 100 pF.
4. Compare spring stiffness k1/k2 of two pendulums with masses of loads of 200g and 400g, respectively, if the periods of their oscillations are equal.
5. Under the action of a stationary load hanging on a spring, its elongation was equal to 6.4 cm. Then the weight was pulled back and released, as a result of which it began to oscillate. Determine the period of these oscillations.
6. A load was suspended from a spring, brought out of its equilibrium position and released. The load began to oscillate with a period of 0.5 s. Determine the elongation of the spring after the oscillations stop. Ignore the mass of the spring.
7. During the same time, one mathematical pendulum makes 25 oscillations, and the other 15. Find their lengths if one of them is 10 cm shorter than the other.8. The oscillatory circuit consists of a capacitor with a capacity of 10 mF and an inductor of 100 mH. Find the amplitude of voltage fluctuations if the amplitude of current fluctuations is 0.1A9. The inductance of the oscillating circuit coil is 0.5 mH. It is required to configure this circuit to a frequency of 1 MHz. What should be the capacitance of the capacitor in this circuit?Exam questions:
1. Which of the following expressions determines the period of free oscillations in an oscillatory circuit? A.; B.  ; IN.
; IN. 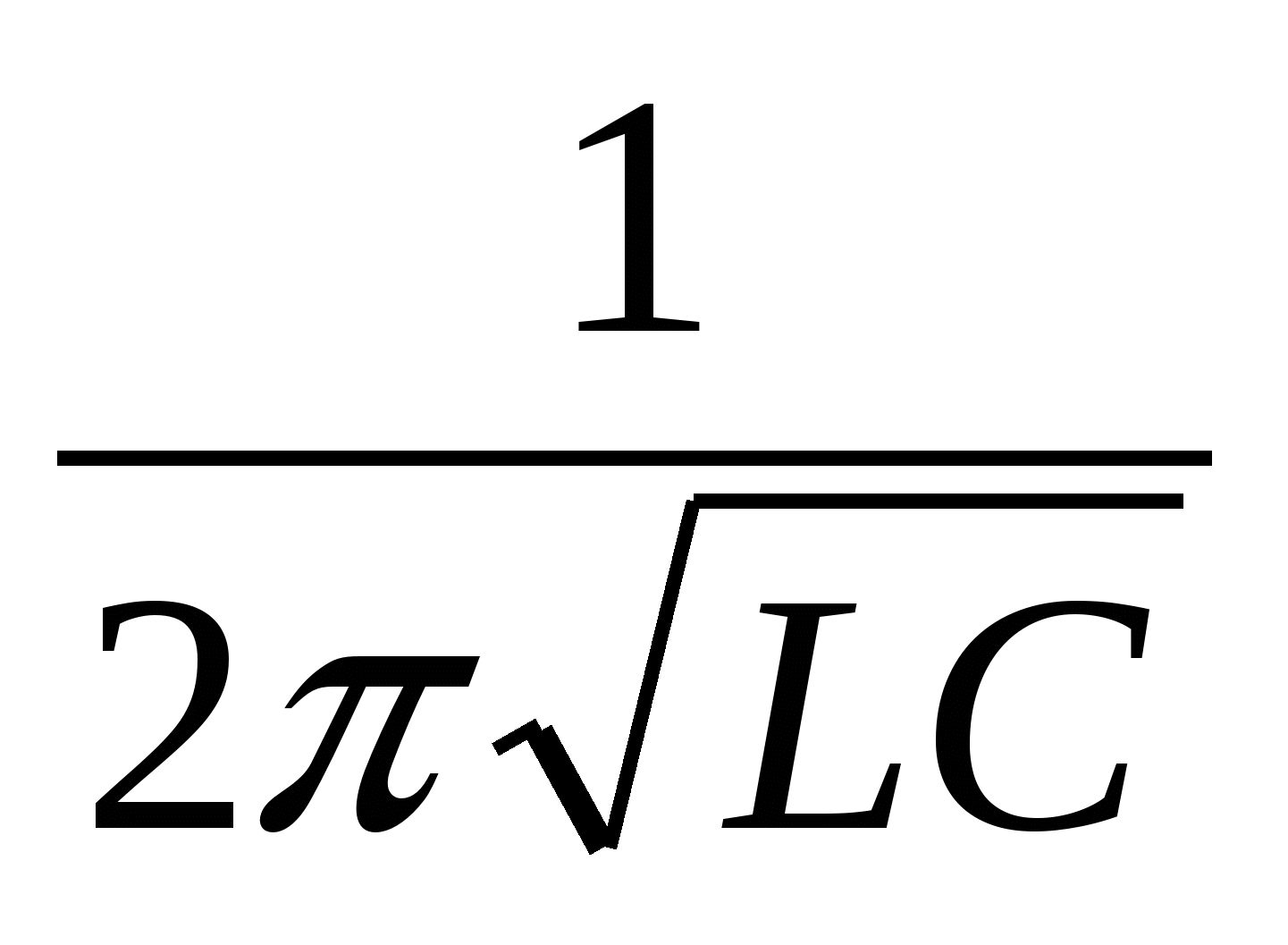 ; G.
; G.  ; D. 2 .
; D. 2 .
2 . Which of the following expressions determines the cyclic frequency of free oscillations in an oscillatory circuit? A.B.
. Which of the following expressions determines the cyclic frequency of free oscillations in an oscillatory circuit? A.B. 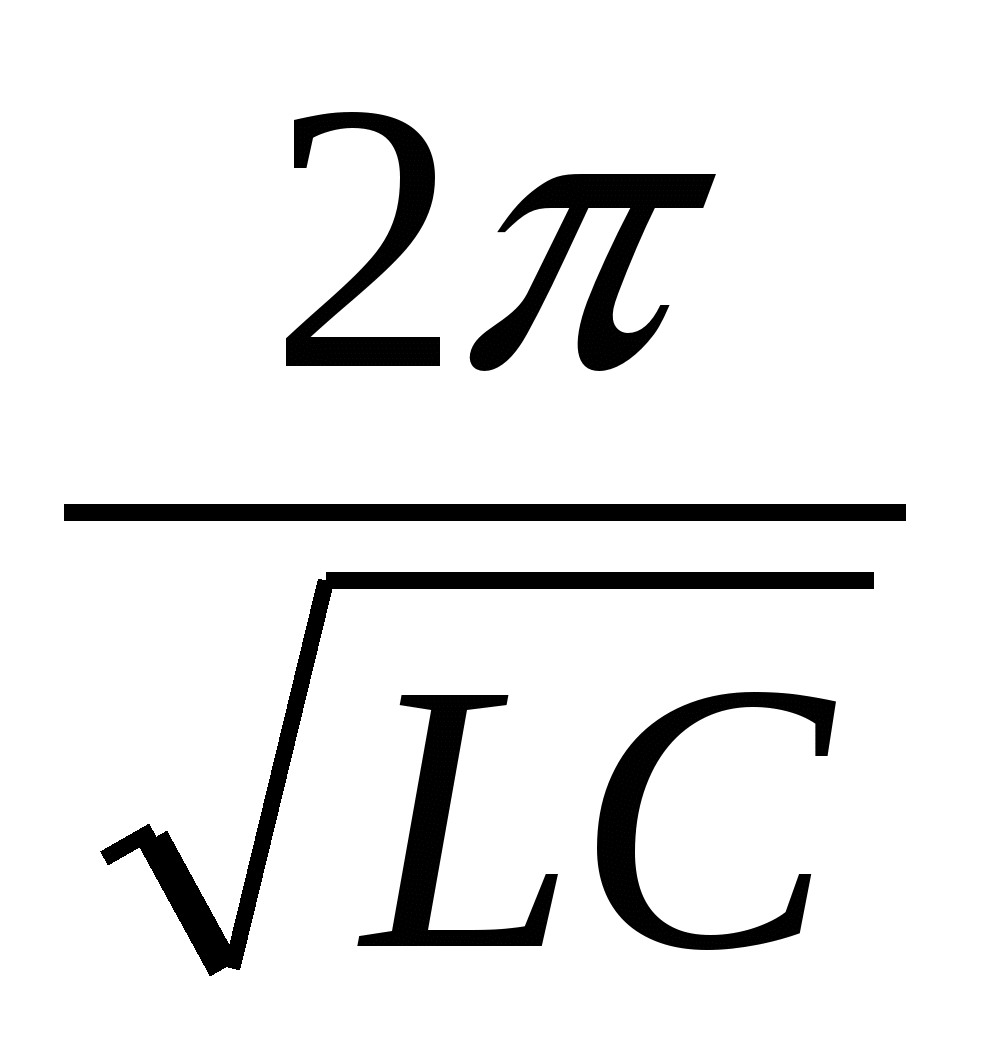 IN.
IN.  G.
G. 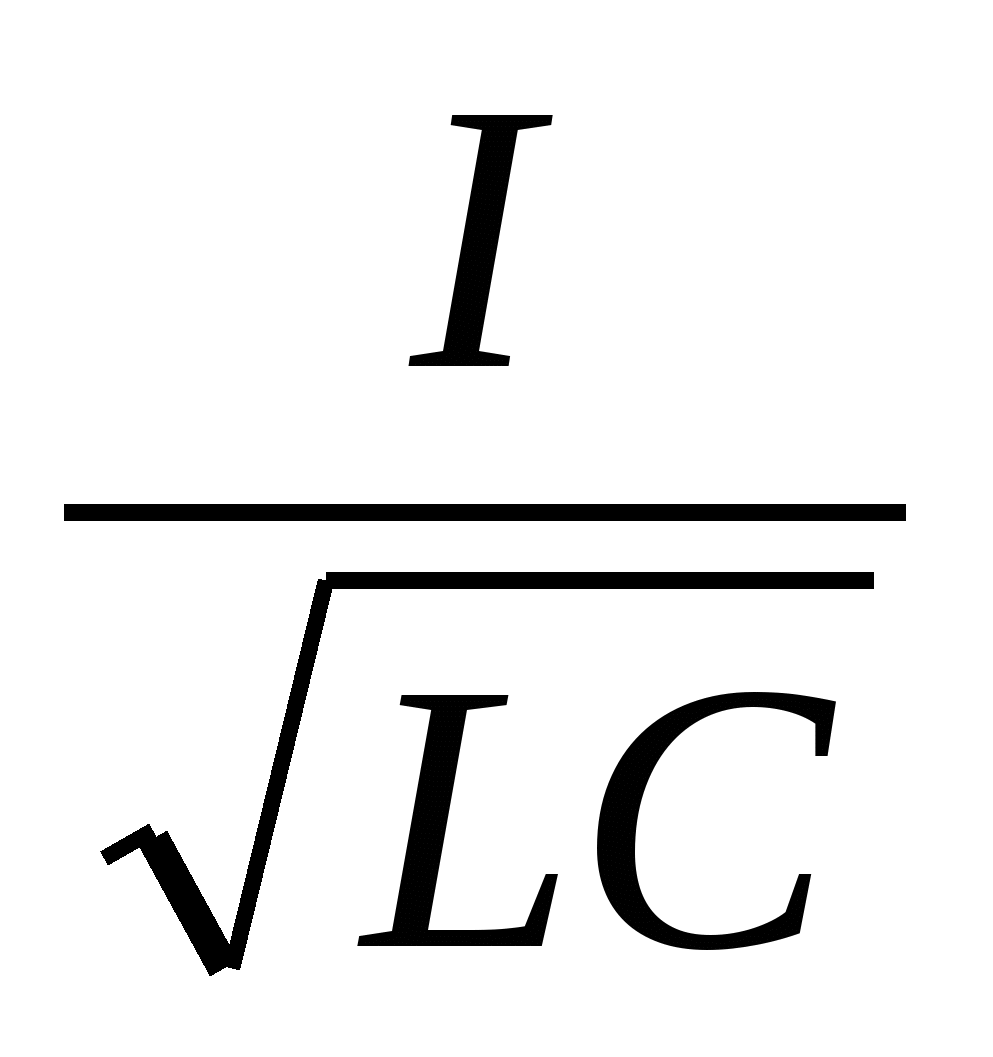 D. 2π
D. 2π
3. The figure shows a graph of the X coordinate of a body performing harmonic oscillations along the x axis as a function of time. What is the period of vibration of the body?
A. 1 s; B. 2 s; V. 3 s . G. 4 p.
4. The figure shows the wave profile at a certain point in time. What is its length?
A. 0.1 m. B. 0.2 m. C. 2 m. D. 4 m. D. 5 m.5
 . The figure shows a graph of the current through the coil of the oscillating circuit versus time. What is the period of current oscillation? A. 0.4 s. B. 0.3 s. V. 0.2 s. G. 0.1 s.
. The figure shows a graph of the current through the coil of the oscillating circuit versus time. What is the period of current oscillation? A. 0.4 s. B. 0.3 s. V. 0.2 s. G. 0.1 s. D. There is no correct answer among answers A-D.

6. The figure shows the wave profile at a certain point in time. What is its length?
A. 0.2 m. B. 0.4 m. C. 4 m. D. 8 m. D. 12 m.
7. Electrical vibrations in the oscillatory circuit are given by the equation q =10 -2 ∙ cos 20t (Cl).
What is the amplitude of charge oscillations?
A . 10 -2 Cl. B.cos 20t Cl. B.20t Cl. G.20 Cl. D. Among the answers A-D there is no correct one.
8. During harmonic vibrations along the OX axis, the coordinate of the body changes according to the law X=0.2cos(5t+  ). What is the amplitude of the body's vibrations?
). What is the amplitude of the body's vibrations?
A. Xm; B. 0.2 m; V. сos(5t+) m; (5t+)m; D.m
9. Oscillation frequency of the wave source is 0.2 s -1 wave propagation speed is 10 m/s. What is the wavelength? A. 0.02 m. B. 2 m. C. 50 m.
D. According to the conditions of the problem, it is impossible to determine the wavelength. D. There is no correct answer among answers A-D.
10. Wave length 40 m, propagation speed 20 m/s. What is the oscillation frequency of the wave source?
A. 0.5 s -1 . B. 2 s -1 . V. 800 s -1 .
D. According to the conditions of the problem, it is impossible to determine the oscillation frequency of the wave source.
D. There is no correct answer among answers A-D.
3
An electromagnetic field can exist in the absence of electric charges or currents: it is these “self-sustaining” electric and magnetic fields that are electromagnetic waves, which include visible light, infrared, ultraviolet and x-ray radiation, radio waves, etc.
§ 25. Oscillatory circuit
The simplest system in which natural electromagnetic oscillations are possible is the so-called oscillatory circuit, consisting of a capacitor and an inductor connected to each other (Fig. 157). Like a mechanical oscillator, for example a massive body on an elastic spring, natural oscillations in the circuit are accompanied by energy transformations.
Rice. 157. Oscillatory circuit
Analogy between mechanical and electromagnetic vibrations. For an oscillatory circuit, an analogue of the potential energy of a mechanical oscillator (for example, the elastic energy of a deformed spring) is the energy of the electric field in a capacitor. An analogue of the kinetic energy of a moving body is the energy of the magnetic field in an inductor. In fact, the energy of the spring is proportional to the square of the displacement from the equilibrium position and the energy of the capacitor is proportional to the square of the charge. The kinetic energy of a body is proportional to the square of its speed and the energy of the magnetic field in the coil is proportional to the square of the current.
The total mechanical energy of the spring oscillator E is equal to the sum of the potential and kinetic energies:
![]()
Energy of vibrations. Similarly, the total electromagnetic energy of the oscillatory circuit is equal to the sum of the energies of the electric field in the capacitor and the magnetic field in the coil:
![]()
From a comparison of formulas (1) and (2) it follows that the analogue of the stiffness k of a spring oscillator in an oscillatory circuit is the reciprocal of the capacitance C, and the analogue of mass is the inductance of the coil
Let us recall that in a mechanical system, the energy of which is given by expression (1), its own undamped harmonic oscillations can occur. The square of the frequency of such oscillations is equal to the ratio of the coefficients of the squares of displacement and speed in the expression for energy:
Natural frequency. In an oscillatory circuit, the electromagnetic energy of which is given by expression (2), its own undamped harmonic oscillations can occur, the square of the frequency of which is also, obviously, equal to the ratio of the corresponding coefficients (i.e., the coefficients of the squares of charge and current):
From (4) follows an expression for the oscillation period, called Thomson’s formula:
![]()
During mechanical oscillations, the dependence of the displacement x on time is determined by a cosine function, the argument of which is called the oscillation phase:
Amplitude and initial phase. The amplitude A and the initial phase a are determined by the initial conditions, i.e., the values of the displacement and velocity at
Similarly, with electromagnetic natural oscillations in the circuit, the charge of the capacitor depends on time according to the law
where the frequency is determined, in accordance with (4), only by the properties of the circuit itself, and the amplitude of charge oscillations and the initial phase a, like that of a mechanical oscillator, are determined
initial conditions, i.e., the values of the capacitor charge and current strength at Thus, the natural frequency does not depend on the method of excitation of oscillations, while the amplitude and initial phase are determined precisely by the excitation conditions.
Energy transformations. Let us consider in more detail energy transformations during mechanical and electromagnetic vibrations. In Fig. 158 schematically depicts the states of mechanical and electromagnetic oscillators at time intervals of a quarter period

Rice. 158. Energy transformations during mechanical and electromagnetic vibrations
Twice during the oscillation period, energy is converted from one type to another and back again. The total energy of the oscillatory circuit, like the total energy of a mechanical oscillator, remains unchanged in the absence of dissipation. To verify this, you need to substitute expression (6) for and expression for the current into formula (2)
Using formula (4) for we obtain

Rice. 159. Graphs of the dependence of the energy of the electric field of the capacitor and the energy of the magnetic field in the coil on the time of charging the capacitor
The constant total energy coincides with the potential energy at the moments when the charge on the capacitor is maximum, and coincides with the energy of the magnetic field of the coil - the "kinetic" energy - at the moments when the charge on the capacitor becomes zero and the current is maximum. During mutual transformations, two types of energy perform harmonic vibrations with the same amplitude, out of phase with each other and with a frequency relative to their average value. This can be easily seen from Fig. 158, and using formulas for trigonometric functions of half an argument:
Graphs of the dependence of the electric field energy and magnetic field energy on the charging time of the capacitor are shown in Fig. 159 for the initial phase
Quantitative laws of natural electromagnetic oscillations can be established directly on the basis of the laws for quasi-stationary currents, without resorting to an analogy with mechanical oscillations.
Equation for oscillations in a circuit. Let's consider the simplest oscillatory circuit shown in Fig. 157. When going around the circuit, for example, counterclockwise, the sum of the voltages on the inductor and capacitor in such a closed series circuit is zero:
![]()
The voltage on the capacitor is related to the charge of the plate and to the capacitance With the relation The voltage on the inductance at any moment of time is equal in magnitude and opposite in sign to the self-inductive emf, therefore the Current in the circuit is equal to the rate of change of the charge of the capacitor: Substituting the current strength in the expression for the voltage on the inductor and denoting the second derivative of the capacitor charge with respect to time through
We obtain Now expression (10) takes the form
![]()
Let's rewrite this equation differently, introducing by definition:
![]()
Equation (12) coincides with the equation of harmonic oscillations of a mechanical oscillator with a natural frequency. The solution to such an equation is given by a harmonic (sinusoidal) time function (6) with arbitrary values of the amplitude and initial phase a. This implies all the above results concerning electromagnetic oscillations in the circuit.
Attenuation of electromagnetic oscillations. So far, natural vibrations in an idealized mechanical system and an idealized LC circuit have been discussed. The idealization consisted of neglecting friction in the oscillator and electrical resistance in the circuit. Only in this case will the system be conservative and the oscillation energy will be conserved.

Rice. 160. Oscillatory circuit with resistance
The dissipation of oscillation energy in the circuit can be taken into account in the same way as was done in the case of a mechanical oscillator with friction. The presence of electrical resistance of the coil and connecting wires is inevitably associated with the release of Joule heat. As before, this resistance can be considered as an independent element in the electrical circuit of the oscillatory circuit, considering the coil and wires ideal (Fig. 160). When considering a quasi-stationary current in such a circuit, the voltage across the resistance must be added to equation (10)
Substituting in we get
Introducing designations
we rewrite equation (14) in the form
Equation (16) for has exactly the same form as the equation for when a mechanical oscillator oscillates with
friction proportional to speed (viscous friction). Therefore, in the presence of electrical resistance in the circuit, electromagnetic oscillations occur according to the same law as the mechanical oscillations of an oscillator with viscous friction.
Dissipation of vibration energy. As with mechanical vibrations, it is possible to establish the law of the decrease in energy of natural vibrations over time by applying the Joule-Lenz law to calculate the heat released:
![]()
As a result, in the case of small attenuation for time intervals much larger than the oscillation period, the rate of decrease in the oscillation energy turns out to be proportional to the energy itself:
![]()
The solution to equation (18) has the form
The energy of natural electromagnetic oscillations in a circuit with resistance decreases according to an exponential law.
The energy of oscillations is proportional to the square of their amplitude. For electromagnetic oscillations this follows, for example, from (8). Therefore, the amplitude of damped oscillations, in accordance with (19), decreases according to the law
Lifetime of oscillations. As can be seen from (20), the amplitude of the oscillations decreases by a factor of a time equal to, regardless of the initial value of the amplitude. This time x is called the lifetime of the oscillations, although, as can be seen from (20), the oscillations formally continue indefinitely. In reality, of course, it makes sense to talk about oscillations only as long as their amplitude exceeds the characteristic value of the level of thermal noise in a given circuit. Therefore, in fact, oscillations in the circuit “live” for a finite time, which, however, can be several times greater than the lifetime x introduced above.
It is often important to know not the lifetime of oscillations x itself, but the number of complete oscillations that will occur in the circuit during this time x. This number multiplied by is called the circuit quality factor.
Strictly speaking, damped oscillations are not periodic. With low attenuation, we can conditionally speak of a period, which is understood as the time interval between two
successive maximum values of the capacitor charge (same polarity), or maximum current values (one direction).
Damping of oscillations affects the period, causing it to increase compared to the idealized case of no damping. With low damping, the increase in the oscillation period is very small. However, with strong attenuation, there may be no oscillations at all: the charged capacitor will discharge aperiodically, i.e., without changing the direction of the current in the circuit. This will happen when i.e. when
Exact solution. The patterns of damped oscillations formulated above follow from the exact solution of differential equation (16). By direct substitution we can verify that it has the form
where are arbitrary constants, the values of which are determined from the initial conditions. At low damping, the cosine multiplier can be considered as a slowly varying amplitude of oscillations.
Task
Recharging capacitors through an inductor. In the circuit, the diagram of which is shown in Fig. 161, the charge of the upper capacitor is equal and the lower one is not charged. At that moment the key is closed. Find the dependence of the charging time of the upper capacitor and the current in the coil.

Rice. 161. At the initial moment of time, only one capacitor is charged

Rice. 162. Charges of capacitors and current in the circuit after closing the key

Rice. 163. Mechanical analogy for the electrical circuit shown in Fig. 162
Solution. After the key is closed, oscillations occur in the circuit: the upper capacitor begins to discharge through the coil, while charging the lower one; then everything happens in the opposite direction. Let, for example, at be the upper plate of the capacitor is positively charged. Then
after a short period of time, the signs of the charges of the capacitor plates and the direction of the current will be as shown in Fig. 162. Let us denote by the charges of those plates of the upper and lower capacitors that are connected to each other through an inductor. Based on the law of conservation of electric charge
The sum of the voltages on all elements of the closed loop at each moment of time is zero:
![]()
The sign of the voltage on the capacitor corresponds to the charge distribution in Fig. 162. and the indicated direction of the current. The expression for the current through the coil can be written in either of two forms:
![]()
Let us exclude from the equation using relations (22) and (24):
![]()
Introducing designations
![]()
Let us rewrite (25) in the following form:
![]()
If instead of entering the function
![]()
and take into account that then (27) takes the form
![]()
This is the usual equation of undamped harmonic oscillations, which has the solution
where and are arbitrary constants.
Returning from the function, we obtain the following expression for the dependence of the charging time of the upper capacitor:
To determine the constants and a, we take into account that at the initial moment the charge and current For the current strength from (24) and (31) we have
Since it follows that Substituting now in and taking into account that we get
![]()
So, the expressions for charge and current have the form

The nature of charge and current oscillations is especially clear when the capacitor capacitances are the same. In this case

The charge of the upper capacitor oscillates with an amplitude around the average value equal to Over half the oscillation period, it decreases from the maximum value at the initial moment to zero, when all the charge is on the lower capacitor.
Expression (26) for the oscillation frequency, of course, could be written right away, since in the circuit under consideration the capacitors are connected in series. However, it is difficult to write expressions (34) directly, since under such initial conditions it is impossible to replace the capacitors included in the circuit with one equivalent one.
A visual representation of the processes occurring here is given by the mechanical analogue of this electrical circuit, shown in Fig. 163. Identical springs correspond to the case of capacitors of the same capacity. At the initial moment, the left spring is compressed, which corresponds to a charged capacitor, and the right one is in an undeformed state, since the analogue of the capacitor charge here is the degree of deformation of the spring. When passing through the middle position, both springs are partially compressed, and in the extreme right position the left spring is undeformed, and the right one is compressed in the same way as the left one at the initial moment, which corresponds to the complete flow of charge from one capacitor to the other. Although the ball undergoes normal harmonic oscillations around its equilibrium position, the deformation of each of the springs is described by a function whose mean value is nonzero.
Unlike an oscillatory circuit with one capacitor, where during oscillations it is repeatedly recharged, in the system under consideration the initially charged capacitor is not completely recharged. For example, when its charge is reduced to zero, and then restored again to the same polarity. Otherwise, these oscillations do not differ from harmonic oscillations in a conventional circuit. The energy of these oscillations is conserved, if, of course, the resistance of the coil and connecting wires can be neglected.
Explain why, from a comparison of formulas (1) and (2) for mechanical and electromagnetic energies, it was concluded that the analogue of stiffness k is and the analogue of mass is inductance and not vice versa.
Provide a rationale for deriving expression (4) for the natural frequency of electromagnetic oscillations in the circuit by analogy with a mechanical spring oscillator.
Harmonic oscillations in a circuit are characterized by amplitude, frequency, period, oscillation phase, and initial phase. Which of these quantities are determined by the properties of the oscillatory circuit itself, and which depend on the method of excitation of oscillations?
Prove that the average values of electric and magnetic energies during natural oscillations in the circuit are equal to each other and constitute half of the total electromagnetic energy of oscillations.
How to apply the laws of quasi-stationary phenomena in an electrical circuit to derive the differential equation (12) of harmonic oscillations in the circuit?
What differential equation does the current in an LC circuit satisfy?
Derive an equation for the rate of decrease in oscillation energy at low damping in the same way as was done for a mechanical oscillator with friction proportional to the speed, and show that for time intervals significantly exceeding the oscillation period, this decrease occurs according to an exponential law. What is the meaning of the term “low attenuation” used here?
Show that the function given by formula (21) satisfies equation (16) for any values of and a.
Consider the mechanical system shown in Fig. 163, and find the dependence on the time of deformation of the left spring and the speed of the massive body.
A circuit without resistance with inevitable losses. In the problem considered above, despite the not entirely ordinary initial conditions for charges on capacitors, it was possible to apply ordinary equations for electrical circuits, since the conditions for quasi-stationary processes were met there. But in the circuit, the diagram of which is shown in Fig. 164, with a formal external similarity to the diagram in Fig. 162, the quasi-stationary conditions are not satisfied if at the initial moment one capacitor is charged and the second is not.
Let us discuss in more detail the reasons why the conditions of quasi-stationarity are violated here. Immediately after closing

Rice. 164. Electric circuit for which quasi-stationary conditions are not met
key, all processes take place only in capacitors connected to each other, since the increase in current through the inductor occurs relatively slowly and at first the branch of the current into the coil can be neglected.
When the key is closed, fast damped oscillations occur in a circuit consisting of capacitors and the wires connecting them. The period of such oscillations is very short, since the inductance of the connecting wires is low. As a result of these oscillations, the charge on the capacitor plates is redistributed, after which the two capacitors can be considered as one. But this cannot be done at the first moment, because along with the redistribution of charges, a redistribution of energy also occurs, part of which turns into heat.
After the fast oscillations decay, oscillations occur in the system, as in a circuit with one capacitor, the charge of which at the initial moment is equal to the initial charge of the capacitor. The condition for the validity of the above reasoning is the smallness of the inductance of the connecting wires compared to the inductance of the coil.
As in the problem considered, it is useful to find a mechanical analogy here. If there two springs corresponding to capacitors were located on both sides of a massive body, then here they should be located on one side of it, so that the vibrations of one of them could be transmitted to the other when the body is stationary. Instead of two springs, you can take one, but only at the initial moment it should be deformed non-uniformly.
Let's grab the spring by the middle and stretch its left half to a certain distance. The second half of the spring will remain in an undeformed state, so that the load at the initial moment is displaced from the equilibrium position to the right by a distance. Under the initial conditions of our problem, when half of the spring is stretched by a distance, the energy reserve is equal to , as is easy to imagine, the stiffness of “half” of the spring is equal to If the mass of the spring is small compared to the mass of the ball, the frequency of natural oscillations of the spring as an extended system is much greater than the frequency of oscillations of the ball on the spring. These “fast” oscillations will die out in a time that is a small fraction of the period of the ball’s oscillations. After the fast oscillations attenuate, the tension in the spring is redistributed, and the displacement of the load remains practically equal since the load does not have time to move noticeably during this time. The deformation of the spring becomes uniform, and the energy of the system equals
Thus, the role of rapid oscillations of the spring was reduced to the fact that the energy reserve of the system decreased to the value that corresponds to the uniform initial deformation of the spring. It is clear that further processes in the system do not differ from the case of uniform initial deformation. The dependence of the displacement of the load on time is expressed by the same formula (36).
In the example considered, as a result of rapid vibrations, half of the initial supply of mechanical energy was converted into internal energy (heat). It is clear that by subjecting not half, but an arbitrary part of the spring to initial deformation, it is possible to convert any fraction of the initial supply of mechanical energy into internal energy. But in all cases, the oscillation energy of the load on the spring corresponds to the energy reserve for the same uniform initial deformation of the spring.
In an electrical circuit, as a result of damped fast oscillations, the energy of a charged capacitor is partially released in the form of Joule heat in the connecting wires. With equal capacities, this will be half the initial energy reserve. The second half remains in the form of energy of relatively slow electromagnetic oscillations in a circuit consisting of a coil and two capacitors C connected in parallel, and
Thus, in this system, idealization in which the dissipation of oscillation energy is neglected is fundamentally unacceptable. The reason for this is that rapid oscillations are possible without affecting the inductor or massive body in a similar mechanical system.
Oscillatory circuit with nonlinear elements. When studying mechanical vibrations, we saw that vibrations are not always harmonic. Harmonic oscillations are a characteristic property of linear systems in which
the restoring force is proportional to the deviation from the equilibrium position, and the potential energy is proportional to the square of the deviation. Real mechanical systems, as a rule, do not possess these properties, and vibrations in them can be considered harmonic only for small deviations from the equilibrium position.
In the case of electromagnetic oscillations in a circuit, one may get the impression that we are dealing with ideal systems in which the oscillations are strictly harmonic. However, this is true only as long as the capacitance of the capacitor and the inductance of the coil can be considered constant, that is, independent of charge and current. A capacitor with a dielectric and a coil with a core, strictly speaking, are nonlinear elements. When a capacitor is filled with a ferroelectric, i.e., a substance whose dielectric constant strongly depends on the applied electric field, the capacitance of the capacitor can no longer be considered constant. Similarly, the inductance of a coil with a ferromagnetic core depends on the current strength, since the ferromagnet has the property of magnetic saturation.
If in mechanical oscillatory systems the mass, as a rule, can be considered constant and nonlinearity arises only due to the nonlinear nature of the acting force, then in an electromagnetic oscillatory circuit nonlinearity can arise both due to a capacitor (analogue of an elastic spring) and due to an inductor ( analogue of mass).
Why is the idealization in which the system is considered conservative not applicable for an oscillatory circuit with two parallel capacitors (Fig. 164)?
Why are rapid oscillations leading to dissipation of oscillation energy in the circuit in Fig. 164, did not occur in a circuit with two series capacitors shown in Fig. 162?
What reasons can lead to non-sinusoidal electromagnetic oscillations in the circuit?
The main device that determines the operating frequency of any alternating current generator is the oscillating circuit. The oscillatory circuit (Fig. 1) consists of an inductor L(consider the ideal case when the coil has no ohmic resistance) and a capacitor C and is called closed. The characteristic of a coil is inductance, it is designated L and measured in Henry (H), the capacitor is characterized by capacitance C, which is measured in farads (F).
Let at the initial moment of time the capacitor be charged in such a way (Fig. 1) that on one of its plates there is a charge + Q 0, and on the other - charge - Q 0 . In this case, an electric field with energy is formed between the plates of the capacitor
where is the amplitude (maximum) voltage or potential difference across the capacitor plates.
After closing the circuit, the capacitor begins to discharge and an electric current flows through the circuit (Fig. 2), the value of which increases from zero to the maximum value. Since a current of variable magnitude flows in the circuit, a self-inductive emf is induced in the coil, which prevents the capacitor from discharging. Therefore, the process of discharging the capacitor does not occur instantly, but gradually. At each moment of time, the potential difference across the capacitor plates
(where is the charge of the capacitor at a given time) is equal to the potential difference across the coil, i.e. equal to the self-induction emf
 |
 |
| Fig.1 | Fig.2 |
When the capacitor is completely discharged and , the current in the coil will reach its maximum value (Fig. 3). The induction of the magnetic field of the coil at this moment is also maximum, and the energy of the magnetic field will be equal to
Then the current begins to decrease, and the charge will accumulate on the capacitor plates (Fig. 4). When the current decreases to zero, the capacitor charge reaches its maximum value Q 0, but the plate, previously positively charged, will now be negatively charged (Fig. 5). Then the capacitor begins to discharge again, and the current in the circuit flows in the opposite direction.
So the process of charge flowing from one capacitor plate to another through the inductor is repeated again and again. They say that in the circuit there are electromagnetic vibrations. This process is associated not only with fluctuations in the amount of charge and voltage on the capacitor, the current strength in the coil, but also with the transfer of energy from the electric field to the magnetic field and vice versa.
 |
 |
| Fig.3 | Fig.4 |
Recharging the capacitor to the maximum voltage will occur only if there is no energy loss in the oscillatory circuit. Such a contour is called ideal.
In real circuits the following energy losses occur:
1) heat losses, because R ¹ 0;
2) losses in the dielectric of the capacitor;
3) hysteresis losses in the coil core;
4) radiation losses, etc. If we neglect these energy losses, then we can write that, i.e.
Oscillations occurring in an ideal oscillatory circuit in which this condition is met are called free, or own, circuit vibrations.
In this case the voltage U(and charge Q) on the capacitor changes according to the harmonic law:
where n is the natural frequency of the oscillatory circuit, w 0 = 2pn is the natural (circular) frequency of the oscillatory circuit. The frequency of electromagnetic oscillations in the circuit is defined as
Period T- the time during which one complete oscillation of the voltage on the capacitor and the current in the circuit occurs is determined Thomson's formula
The current strength in the circuit also changes according to the harmonic law, but lags behind the voltage in phase by . Therefore, the dependence of the current strength in the circuit on time will have the form
![]() . (9)
. (9)
Figure 6 shows graphs of voltage changes U on the capacitor and current I in the coil for an ideal oscillating circuit.
In a real circuit, the energy will decrease with each oscillation. The amplitudes of the voltage on the capacitor and the current in the circuit will decrease; such oscillations are called damped. They cannot be used in master oscillators, because The device will work at best in pulse mode.
 |
 |
| Fig.5 | Fig.6 |
To obtain undamped oscillations, it is necessary to compensate for energy losses at a wide variety of operating frequencies of devices, including those used in medicine.
Thomson's formula named after the English physicist William Thomson, who derived it in 1853, and connects the period of natural electrical or electromagnetic oscillations in a circuit with its capacitance and inductance.Thomson's formula is as follows:
see also
Write a review about the article "Thomson's Formula"
Notes
Excerpt characterizing Thomson's Formula
- Yes, yes, I know. Let’s go, let’s go...” said Pierre and entered the house. A tall, bald old man in a dressing gown, with a red nose, and galoshes on his bare feet, stood in the hallway; Seeing Pierre, he muttered something angrily and went into the corridor.“They were of great intelligence, but now, as you can see, they have weakened,” said Gerasim. - Would you like to go to the office? – Pierre nodded his head. – The office was sealed and remains so. Sofya Danilovna ordered that if they come from you, then release the books.
Pierre entered the same gloomy office that he had entered with such trepidation during the life of his benefactor. This office, now dusty and untouched since the death of Joseph Alekseevich, was even gloomier.
Gerasim opened one shutter and tiptoed out of the room. Pierre walked around the office, went to the cabinet in which the manuscripts lay, and took out one of the once most important shrines of the order. These were genuine Scottish deeds with notes and explanations from the benefactor. He sat down at a dusty desk and put the manuscripts in front of him, opened them, closed them, and finally, moving them away from him, leaning his head on his hands, began to think.